In an acute triangle, the square of the side opposite to an acute angle is equal to the sum of the squares of other two sides minus twice the product of one side and the projection of the other on first.

Solution :

Given : In triangle ABC angle B is an acute angle and AD \(\perp\) BC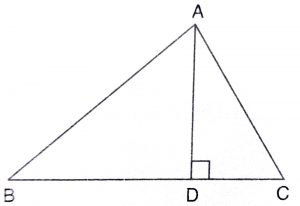

To Prove : \({AC}^2\) = \({AB}^2\) + \({BC}^2\) – 2BC.BD

Proof : Since \(\triangle\) ADC is a right triangle, angled at D. Therefore, by Pythagoras theorem,

\({AC}^2\) = \({AD}^2\) + \({CD}^2\)

\(\implies\)  \({AC}^2\) = \({AD}^2\) + \({BC – BD}^2\)                   (because DC = BC – BD)

\(\implies\)  \({AC}^2\) = \({AD}^2\) + \({BC}^2\) + \({BD}^2\) – 2BC.BD

Since, In triangle ADB, \({AB}^2\) = \({AD}^2\) + \({BD}^2\)

\(\implies\)  \({AC}^2\) = \({AB}^2\) + \({BC}^2\) – 2BC.BD