What is the Value of Cos 60 Degrees ?

Solution :

The value of Cos 60 degrees is $1\over 2$.

Proof :

Consider an equilateral triangle ABC with each side of length of 2a. Each angle of $\Delta$ ABC is of 60 degrees. Let AD be the perpendicular from A on BC.

$\therefore$   AD is the bisector of $\angle$ A and D is the mid-point of BC.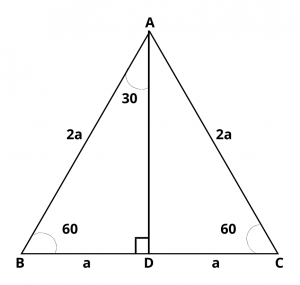

$\therefore$   BD = DC = a and $\angle$ BAD = 30 degrees.

In $\Delta$ ADB, $\angle$ D is a right angle, AB = 2a and BD = a

By Pythagoras theorem,

$AB^2$ = $AD^2$ + $BD^2$  $\implies$  $2a^2$ = $AD^2$ + $a^2$

$\implies$  $AD^2$ = $4a^2$ – $a^2$ = $3a^2$   $\implies$  AD = $\sqrt{3}a$

Now, In triangle ADB, $\angle$ B = 60 degrees

By using trigonometric formulas,

$cos 60^{\circ}$ = $base\over hypotenuse$ = $b\over h$

$cos 60^{\circ}$ = side adjacent to 60 degrees/hypotenuse = $BD\over AB$ = $a\over 2a$ = $1\over 2$

Hence, the value of $cos 60^{\circ}$ = $1\over 2$