Solution :
The general solution of cosθ = 0 is given by θ = (2n+1)π2, n ∈ Z.
Proof :
We have,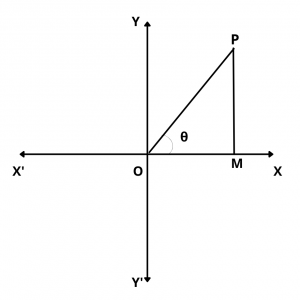
cosθ = PMOP
∴ cosθ = 0
⟹ OMOP = 0
⟹ OM = 0
⟹ OP coincides with OY or OY’
⟹ θ = ±π2, ±3π2, ±5π2, …….
⟹ θ = (2n+1)π2, n ∈ Z
Hence, θ = (2n+1)π2, n ∈ Z is the general solution of cosθ = 0.