Into Function – Definition and Examples

Here you will learn what is into function with definition and examples.

Let’s begin –

Into Function Definition

Definition : A function f : A $\rightarrow$ B is said to be an into function if every element of B having no pre-image in A.

In other words, f : A $\rightarrow$ B is an into function if it is not an onto function.

Also Read : Types of Functions in Maths – Domain and Range

Example : Let A $\rightarrow$ B be the function represented by the following diagram :

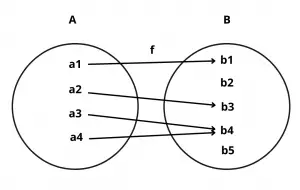
Solution : Clearly, b2 and b5 are two elements in B which do not have their pre-images in A. So, f : A $\rightarrow$ B is an into function.

Example : A function f : N $\rightarrow$ N defined by f(x) = 2x is into function, because f(N) = {2, 4, 6, ….} $\ne$ N (co-domain). In other words, range (f) $\ne$ co-domain of f.

Example : Let f : R $\rightarrow$ R given by f(x) = $x^2 + 2$ for all x $\in$ R. Then, find whether it is into or not.

Solution : Clearly, f(x) = $x^2 + 2$ $\ge$ 2 for all x $\in$ R. So, negative real numbers in R (domain) do not have their pre-images in R (domain).

Hence, f is an into function.