Volume of a Frustum of a Cone – Formula and Derivation

Here you will learn formula for the volume of a frustum of a cone with derivation and examples based on it.

Let’s begin – 

What is Frustum of Cone ?

Frustum of a cone is the solid obtained after removing the upper portion of the cone by a plane parallel to its base. The lower portion is the frustum of a cone.

Height : The perpendicular distance between the aforesaid plane and the base is called the height of the frustum.

Volume of a Frustum of a Cone

The formula for volume of a frustum of a cone is

V = $h\over 3$$[A_1 + \sqrt{A_1A_2} + A_2]$

[$A_1$, $A_2$ are the areas of bottom and top of the frustum]

V = $\pi h\over 3$$[{r_1}^2 + \sqrt{r_1r_2} + {r_2}^2]$

where h is the height of the frustum, $r_1$, $r_2$ are the radii of the base and the top of frustum of a cone.

Note : Height h of the frustum is given by the relation,

$l^2$ = $h^2$ + $(r_1 – r_2)^2$

Also Read : Area of a Frustum of a Cone – Formula and Derivation

Derivation :

Let $r_1$ and $r_2$ be the radii of the bottom and top of the frustum respectively and h be the height of the frustum.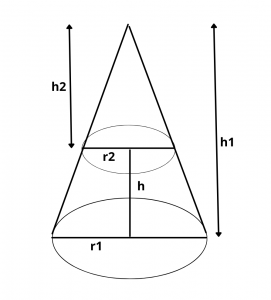

The frustum of a cone is the solid obtained after removing the upper portion (small cone) of it by a plane parallel to the base of the big cone.

Volume of frustum = Volume of bigger cone – Volume of smaller cone

= $1\over 3$$\pi {r_1}^2 h_1$ – $1\over 3$$\pi {r_2}^2 h_2$

Since, [ $h_1\over r_1$ = $h_2\over r_2$ = k ]

= $\pi k\over 3$$[{r_1}^3 – {r_2}^3]$

= $\pi k\over 3$ ($r_1 – r_2$) $({r_1}^2 + {r_1r_2} + {r_2}^2)$

Volume = $1\over 3$ ($kr_1 – kr_2$) $(\pi{r_1}^2 + \pi{r_1r_2} + \pi{r_2}^2)$

V = $1\over 3$ ($h_1 – h_2$) $(\pi{r_1}^2 + \sqrt{(\pi{r_1}^2)(\pi{r_2}^2)} + \pi{r_2}^2)$

Volume = $h\over 3$$[A_1 + \sqrt{A_1A_2} + A_2]$

Where $A_1$, $A_2$ are the areas of bottom and top of the frustum respectively and h = $h_1 – h_2$.

Volume of frustum of Cone = $h\over 3$$[A_1 + \sqrt{A_1A_2} + A_2]$

= $h\over 3$ $(\pi{r_1}^2 + \sqrt{(\pi{r_1}^2)(\pi{r_2}^2)} + \pi{r_2}^2)$

Volume  = $\pi h\over 3$$[{r_1}^2 + \sqrt{r_1r_2} + {r_2}^2]$

Example : A friction clutch is in the form of the frustum of a cone, the diameters of the ends being 8 cm, and 10 cm and length 8 cm. Find its Volume.

Solution : Here, radius, 

$r_1$ = $10\over 2$ = 5cm,

$r_2$ = $8\over 2$ = 4cm

Slant height, l = 8 cm

Height h of the frustum is given by the relation,

$l^2$ = $h^2$ + $(r_1 – r_2)^2$

$\implies$  64 = $h^2$ + $(5 – 4)^2$  or  $h^2$ = 63  $\implies$ h = 7.937

$\therefore$  Volume = $\pi h\over 3$$[{r_1}^2 + \sqrt{r_1r_2} + {r_2}^2]$

Volume = $3.14 \times 7.937\over 3$ $\times$ [25 + 20 + 16] = 506.75 $cm^2$